User:Lothar.brendel: Difference between revisions
Jump to navigation
Jump to search
2D flow in polar coordinates
(→Scratch Pad: +Hamiltons) |
(→Windows 11: local account) |
||
| (6 intermediate revisions by the same user not shown) | |||
| Line 9: | Line 9: | ||
* vorticity: | * vorticity: | ||
* its gradient: | * its gradient: | ||
== Windows 11 == | |||
* https://www.howtogeek.com/836157/how-to-use-windows-11-with-a-local-account | |||
== Energy equation == | == Energy equation == | ||
| Line 22: | Line 26: | ||
\partial_t E' &= -\vec\nabla\cdot\big((E'+P)\vec u\big)+\vec u\cdot\vec f | \partial_t E' &= -\vec\nabla\cdot\big((E'+P)\vec u\big)+\vec u\cdot\vec f | ||
\end{align} | \end{align} | ||
== Florian's eCDF == | |||
Let | |||
$$ | |||
c(n_i)-c(n_{i-1})\stackrel{*}{=}c(n_i)-c(n_i-1)=c(n_i)-c(n_i-\epsilon)=p_{n_i}~. | |||
$$ Florian's modified eCDF replaces the step-function | |||
$$ | |||
s_{n_i}=\frac{\tilde c(n_i)-\tilde c(n_{i-1})}{n_i-n_{i-1}} = \frac{c(n_i)-c(n_{i-1})}{n_i-n_{i-1}}~, | |||
$$ | |||
which implies | |||
$$ | |||
\sum_{n=n_{i-1}+1}^{n_i} \tilde p_n = (n_{i}-n_{i-1})\tilde p_n = (n_{i}-n_{i-1})s_{n_i} = c(n_i)-c(n_{i-1}) = p_{n_i}~. | |||
$$ | |||
Hence, the effect of using | |||
<nowiki>*</nowiki>: even though in general | |||
== Hamiltons principle == | == Hamiltons principle == | ||
| Line 27: | Line 49: | ||
* Phys.SE: [https://physics.stackexchange.com/questions/560216/modified-hamiltons-principle-overconstraining-a-system-by-imposing-too-many-bou Modified Hamilton's Principle overconstraining a system by imposing too many boundary conditions] | * Phys.SE: [https://physics.stackexchange.com/questions/560216/modified-hamiltons-principle-overconstraining-a-system-by-imposing-too-many-bou Modified Hamilton's Principle overconstraining a system by imposing too many boundary conditions] | ||
* Phys.SE: [https://physics.stackexchange.com/questions/431685/boundary-conditions-for-calculus-of-variations-in-phase-space-and-under-canonica Boundary conditions for calculus of variations in phase space and under canonical transformations] | * Phys.SE: [https://physics.stackexchange.com/questions/431685/boundary-conditions-for-calculus-of-variations-in-phase-space-and-under-canonica Boundary conditions for calculus of variations in phase space and under canonical transformations] | ||
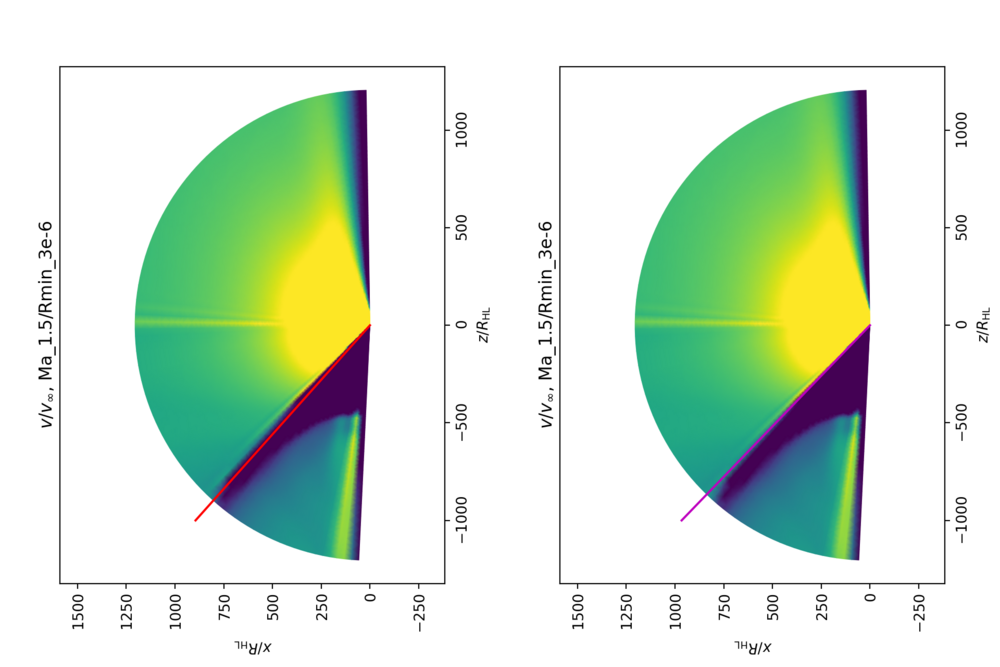
== Mach-Angle == | |||
[[File:Vovoo Ma1.5 Rmin3e-6.png|1000px|normalized velocity, strongly focused around unity]] | |||
Latest revision as of 12:00, 13 March 2025
Lothar Brendel
"Admin" of this Wiki
Scratch Pad
2D flow in polar coordinates
- vorticity:
- its gradient:
Windows 11
Energy equation
Florian's eCDF
Let
*: even though in general
Hamiltons principle
- Phys.SE: Modified Hamilton's Principle overconstraining a system by imposing too many boundary conditions
- Phys.SE: Boundary conditions for calculus of variations in phase space and under canonical transformations